Black’s Model for Swaption

The quoting convention of the swaption volatility is annualized normal vol under annuity measure. Here, normal is contrary to log-normal. It is also called Black’s (vs Balck-Scholes) or Bachelier’s model. We will use formulas to explain what it means.

Note 1: Black’s model is used for quoting only in modern financial modeling. It doesn’t mean the dynamics of interest rates are modeled this way. A commonly used model for swaption is SABR (stochastic alpha beta rho).

Note 2: The formula below ignores nuances of the effective date, settlement date, and day count convention.

Notations:

$\hat{T}$ : maturity of swaption

$\Delta$ : tenor (maturity) of the underlying swap

$\mathbb{Q}$ : risk neutral measure

$A$ : annuity or annuity measure where annuity matches the floating leg pay frequency of the underlying swap

$K$ : strike

$P(0,t)$ : discount factor from $t (t \ge 0)$ to $0$

$S_{t}(\hat{T}, \hat{T}+\Delta)$ : par swap rate for a swap contract from $\hat{T}$ to $\hat{T}+\Delta$ seen at $t$ . We define a short-hand $S_t := S_t(\hat{T} - \hat{T}+\Delta)$ when there is no confusion.

$\mathbb{E}_t^{\mathbb{P}}$ : conditional expectation on $\mathcal{F}_t$ for filtration $(\Omega,\mathcal{F}, (\mathcal{F}_t)_{t\ge 0},\mathbb{P})$

$PV_{\mbox{something}}$ : present value of something.

$V_{\mbox{something}}(t)$ : value of something at time $t$ .

Below, we set up the formula for PV of a payer swaption assuming the notional is $1. For a more general notional, just scale the formula up by the notional. In a payer swaption, the purchaser has the right but not the obligation to enter into a swap contract where they become the fixed-rate payer and the floating-rate receiver.

$\small \begin{aligned} PV_{\mbox{payer}} =& \mathbb{E}_0^{\mathbb{Q}}\left[\left(V_{\mbox{float}}(\hat{T})-V_{\mbox{fixed}}(\hat{T})\right)^+P(0,\hat{T})\right] \\ =& \mathbb{E}_0^{\mathbb{Q}}\left[ \left(S_{\hat{T}}(\hat{T},\hat{T}+\Delta)A(\hat{T})-KA(\hat{T})\right)^+P(0,\hat{T})\right] \\ = & A(0) \mathbb{E}^{A}_{0} \left[\left(S_{\hat{T}}(\hat{T},\hat{T}+\Delta) - K\right)^+\right] \end{aligned}$

The last line of the above formula is a change of numeraire from risk-neutral measure to annuity measure.

Since swaption vol is quoted as Bachelier’s vol in annuity measure, the dynamic of interest rate under this measure is the following:

$\small \textrm{d} S_t = \sigma \textrm{d} W_t,$

where $W_t$ is a standard Brownian motion under annuity measure and $\sigma$ is constant.

Hence (by integrating both sides from $0$ to $\hat{T}$ ), denoting by $\mathcal{N}(\mbox{mean}, s.d.)$ a normal distribution, we have

$\small S_{\hat{T}} \sim \mathcal{N}(S_0, \sigma\sqrt{\hat{T}}).$

After plugging in the Gaussian density, the annuity measure expectation becomes

$\small \begin{aligned} & \mathbb{E}^{A}_{0} \left[\left(S_{\hat{T}} - K\right)^+\right] \\ = & \int_{K}^{+\infty} (x-K) \frac{1}{\sigma \sqrt{2\pi\hat{T}}} e^{-\frac{(x-S_0)^2}{2\sigma^2\hat{T}}} \textrm{d} x \\ = & \sigma \sqrt{\hat{T}} \cdot \varphi (d_1) + (S_0 - K) \cdot \Phi(d_1) \end{aligned} ,$

where

$\varphi$ is the probability density function of the standard Gaussian distribution

$\Phi$ is the cumulative density function of the standard Gaussian distribution

$d_1 := \frac{S_0 - K}{\sigma \sqrt{\hat{T}}}.$

Finally,

$\small PV_{\mbox{payer}} = A(0) \left[\sigma \sqrt{\hat{T}} \cdot \varphi (d_1) + (S_0 - K) \cdot \Phi(d_1) \right]$

The Greeks are as follows:

Delta = $A(0) \Phi(d_1)$

Vega = $A(0) \varphi(d_1) \sqrt{\hat{T}}$

Gamma = $A(0)\frac{\varphi(d_1)}{\sigma \sqrt{\hat{T}}}$

The vega-gamma relationship is:

$\small \mbox{Vega} =\hat{T}} \sigma \mbox{Gamma}$

The value of annuity $A(0)$ depends on discounting curve, but one can approximate it by tenor ( $\Delta$ ) in years, i.e., no discounting. The swaption Bachelier’s/normal/Black’s vol is quoted in the unit of bps. For example, 107 means 107bps, i.e., 0.0107. Below we provide two examples of PV: one ATM and one ITM.

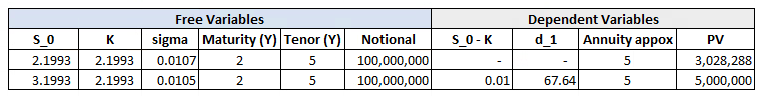
For a receiver swaption, PV and greeks can be calculated in the same manner.