Finance – Page 3 – Sisi Tang

March 8, 2019March 8, 2021

European call option v.s. Asian call option

Let a stock follow a Geometric Brownian motion

$\textnormal{d}S_t = S_t(r \textnormal{d} t + \sigma \textnormal{d} W_t)$

with constant $r,\sigma > 0$ . Let

$A_T = \frac{1}{T}\int_0^T S_u \textnormal{d} u$

European Asian call option has the payoff $(A_T-K)^+$ and European vanilla call option has the payoff $(S_T-K)^+$ . Then European vanilla option has higher value.

Proof.

By (1)Jensen’s inequality, (2)Fubini, and (3)the fact that the longer the maturity is the higher the vanilla European option’s value is, we have

$\begin{aligned} &~ \mathbb{E}[e^{-rT}(A_T-K)^+] \\= &~ \mathbb{E}[e^{-rT}(\frac{1}{T}\int_0^T S_u \textnormal{d} u -K)^+] \\\leq & ~ \mathbb{E}[e^{-rT}\frac{1}{T}\int_0^T (S_u-K)^+ \textnormal{d} u] \\= &~ \frac{1}{T}\int_0^T \mathbb{E}[e^{-rT} (S_u-K)^+ ] \textnormal{d} u \\\leq &~ \frac{1}{T}\int_0^T \mathbb{E}[e^{-ru} (S_u-K)^+ ] \textnormal{d} u \\\leq &~ \frac{1}{T}\int_0^T \mathbb{E}[e^{-rT} (S_T-K)^+ ] \textnormal{d} u \\ = &~ \mathbb{E}[e^{-rT} (S_T-K)^+ ] \\ \end{aligned}$

Notice that we didn’t use the dynamics of Geometric Brownian motion. Above deduction is true as long as (3) is true.

February 4, 2019March 8, 2021

Cross Hedging

When our study group read John Hull’s Options, Futures, and Other Derivatives 10th Edition book section 3.4 Cross Hedging, the hedging ratio $h^*$ was given directly in (3.1). We filled in the derivation of it here.

Goal

We want to hedge an asset using a future contract whose underlying asset is different from the one being hedged. This usually happens for example in the case that the asset being hedged is not available in the future market. If we are going to sell an asset, then we take long position in a future contract; short otherwise.

Setting

$h$ : hedge ratio. The ratio of the size of the position taken in futures contracts to the size of the exposure.
$\Delta S$ : change of spot price of the asset to be hedged.
$\Delta F$ : change of price in the future contract.

Formulation

Mathematically, we want to minimize the variance of our portfolio value, i.e.,

$\begin{aligned}\mbox{Var}(\Delta S-h\Delta F) &= \mathbf{E}[(\Delta S-\overline{\Delta S}-h(\Delta F-\overline{\Delta F}))^2] \\&= \mathbf{E}[(\Delta S-\overline{\Delta S})^2] + h^2\mathbf{E}[(\Delta F-\overline{\Delta F})^2] \\ & \quad - 2h\mathbf{E}[(\Delta S-\overline{\Delta S})(\Delta F-\overline{\Delta F})] \\&= \sigma_{\Delta S}^2+ h^2\sigma_{\Delta F}^2 - 2h~\mbox{cov}(\Delta S,\Delta F) ,\end{aligned}$

where $\sigma_{\Delta S}, \sigma_{\Delta F}$ denote the standard deviation of $\Delta S$ , standard deviation of $\Delta F$ respectively. This is a quadratic fucntion in $h$ . The minimum of it is achieved at

$h=\frac{\mbox{cov}(\Delta S,\Delta F)}{\sigma_{\Delta F}^2}=\rho\frac{\sigma_{\Delta S}}{\sigma_{\Delta F}},$

where $\rho$ is the correlation of $\Delta S$ and $\Delta F$ . This formula for $h$ is exactly the slope of linear regression $\Delta S \sim \Delta F$ . Our derivation here also explains why linear regression is the minimum variance estimator.

Then, the variance

$\mbox{Var}(\Delta S-h\Delta F)=(1-\rho^2)\sigma_{\Delta S}^2.$

Hedge effectiveness: the proportion of the variance that is eliminated by hedging.
The hedge effectiveness in our case is

$\eta = 1-\frac{\mbox{Var}(\Delta S-h\Delta F)}{\mbox{Var}(\Delta S)} = \rho^2,$

which is the $R^2$ of the linear regression $\Delta S \sim \Delta F$ .

Conclusion

The hedging ratio is

$h^*=\frac{\mbox{cov}(\Delta S,\Delta F)}{\sigma_{\Delta F}^2}=\rho\frac{\sigma_{\Delta S}}{\sigma_{\Delta F}}.$

Hedge effectiveness is $\rho^2$ .

February 3, 2019March 8, 2021

Bond Yield v.s. Bond Price

When our study group read John Hull’s Options, Futures, and Other Derivatives book section 4.10 Duration, there was a sentence that is not very intuitive: There is a negative relationship between bond yield and bond price. (When bond yields decrease, bond prices increase. When bond yields increase, bond price decrease.)

Intuitively, people would believe a high “yield” bond has high price. Here is an explanation to the anti-intuition fact.

Assumption
Suppose the coupon rate and time of payments of a bond are fixed. (It is fixed the time it is designed.) Namely, at time 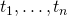$t_1, \ldots, t_n$ , the bond holder will receive 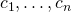$c_1, \ldots, c_n$ dollars. The last payment 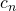$c_n$ contains the
notional/face value of the bond. At time 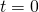$t=0$ , maket has a quote 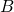$B$ of this bond, i.e., how much people are willing to pay for this bond.

Formulation
Bond yield 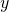$y$ is defined as the discount rate such that the sum of the present values of all payments is equal to the bond price 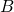$B$ . That is to say, 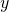$y$ is solved from the following equation:

$B = \sum_{i=1}^n c_ie^{-yt_i}$

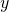
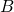
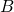
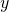
In the above equation, there are only two variables $y$ and $B$ . Hence, $B$ is a function of $y$ and vice versa.

$B=B(y)$

Then,

$\mathrm{d}B = \frac{ \mathrm{d} B}{ \mathrm{d} y} \mathrm{d}y.$

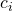
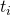
No partial derivatives because $c_i$ and $t_i$ are fixed. They are not variables.

Approximately, small change 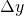$\Delta y$ in bond yield would result in the change of the bond price being approximately

$\Delta B = \frac{ \mathrm{d} B}{ \mathrm{d} y} \Delta y.$

That is,

$\Delta B = -\sum_{i=1}^n c_it_ie^{-yt_i} \Delta y.$

Duration of a bond is defined to be the weighted average of the time of coupon payments and notional, where the weight is the present value. Namely,

$D := \sum_{i=1}^n t_i\left[\frac{c_ie^{-yt_i}}{B}\right]$

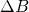
Plug this into the equation of $\Delta B$ , we get

$\Delta B = - BD \Delta y.$

Explanation
Mathematically, if 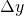$\Delta y$ is positive, then 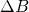$\Delta B$ is negative. If 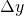$\Delta y$ is negative, then 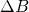$\Delta B$ is positive.
Intuitively, bond yield and bond price are two ways to quote a bond; just like in describing fuel efficiency, you can use miles/gallon or liters/hundred miles. There is a one to one correspondence between bond yield and bond price. Higher bond yield is corresponding to a lower bond price. We can not say higher bond yield results in lower bond price.

The usual “yield” in people’s mind is actually coupon rate. Higher coupon rate definitely results in higher bond price (if other conditions keep the same).

The market usually quotes bond yield. People can get the approximated bond price change by the formula $\Delta B = - BD \Delta y$ .

What causes the change of a bond price?
Change of interest rate (risk-free rate).
If the interest rate rockets, then the present value of a payment in the future will worth less money. Then, th bond price, which is the sum of present values of all payments in the future, will decrease.